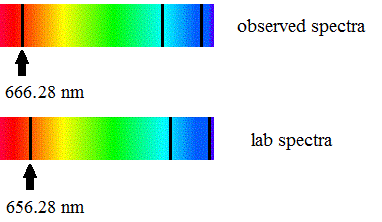
An astronomer observes the hydrogen spectra of an object and compares it to a similar hydrogen spectra found in the lab. From this, it is possible to calculate radial velocity of the object. Can you? The numbers represent the wavelength (measured in nanometers) of one of the spectral lines found in hydrogen. Hint: You will need to find and use the appropriate equation necessary to obtain the answer. Please show all your work.
Make sure you indicate if the object is approaching or receding as well as a numerical answer with proper units.
Bonus points if you can give the value of "z" which is a unit-less number used to indicate the Doppler shift.
ANSWER:
You first need to find the necessary equation to obtain the radial velocity. It can be found in many forms. Below is the simplest form and all you need:
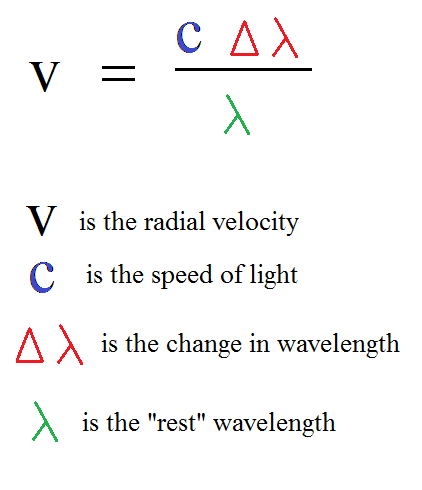
Let's use c (speed of light) = 299,792,458 m / s
Δ
= 10 nm
Note: Subtract 666.28 - 656.28 = 10 nm
= 656.28 nm
This is the "rest" wavelength of the spectral line.
v = (299,792,458 m/s) (10 nm) / (656.28 nm) = 4,568,000 m/s This is a (pretty big) red shift so the object is moving away from us. Notice that the nm units cancel out leaving the units in m/s.
Bonus points: z is a ratio defined as:
Δ
/ using the same
definitions as above. Hence, z = 10 nm / 656.28 nm = .015 (the
nm units cancel out leaving a unit-less value)
Additional note: The famous astronomer, Edwin Hubble was doing these kinds of calculations for galaxies and coming up with similar values. From the numbers he concluded that the universe was expanding. This was really big news in the 1920's. Not only that, but Hubble noticed that the greater the redshift, the further the galaxy was from us. Based in current values, this object would have to be a couple hundred million light years away from us. This page actually does the calculation for you. Plug in a value z = .015 and it shows (down the page) that the object would be 206 million light years away based on Hubble's Law of Redshifts (something we discuss in unit 5).