Compressions and rarefactions in air make sound (animation)
Key Terms
frequency
human ear
resonance
Matter vibrates! Atoms vibrate ... molecules vibrate ... solids vibrate ... the whole earth vibrates. When things vibrate, they can start a chain reaction. The oscillations of the object can strike air molecules and transport some of that energy away from the object in the form of sound waves. Those waves can, in turn, interact with other forms of matters and make them vibrate. The rate at which this happens determines the frequency of the oscillations. That is, how many times a second it wiggles back and forth. The unit for frequency is the hertz (Hz), and can be replaced with "oscillations per second."

Compressions and rarefactions in air make sound (animation)
When an object vibrates (like a tuning fork) it sets up tiny waves of pressure in the air we detect as sound. Sound waves are called compression waves (or longitudinal waves) because the air gets compressed by the vibrating object and that compression is in the same direction as the wave travels. Between the compressions, the air is spread apart (lower pressure) and is typically called rarefactions. The number of compression waves that pass by your ear is equal to the rate of the tuning fork oscillations ... that is, they have the same frequency.

Animation of a compression wave
Musical instruments (like the piano or acoustic guitar) make use of the vibrations of wires. As the wires are struck or plucked and forced to vibrate, they set up similar vibrations in the air, which we pick up as sounds of different pitches. The frequency of waves is determined by the tension in the wires. The higher the tension in the wire, the higher the pitch of the sound produced.
This can be demonstrated by listening to tuning forks of different lengths. If the tuning fork is rated at 1000 hertz, that means it will vibrate naturally at 1000 times a second (its frequency) and produce a sound with a distinct pitch.
Click link 6.2.a to hear a tone frequency of 1000 hertz (waves per second).
Click link 6.2.b to hear assorted frequencies.
Sound vibrations moves through air at about 761 mph, or 1100 feet/second (at sea level).
The human ear can detect sound frequencies between the range 20 Hz - 20 kHz (20 - 20,000 waves per second). Your ears are also incredibly sensitive, with the ability to detect pressure variations less than a billionth of atmospheric pressure.
The ear consists of 3 sections - outer ear, middle ear, and inner ear.
The outer ear is the collector - which acts like a funnel to direct and amplify the sound waves to the middle ear. Once in the middle ear, the waves strike the eardrum. The eardrum then vibrates with the same frequency as the incoming waves. The vibrations of the eardrum are transported to the inner ear by 3 tiny bones (hammer, anvil, and stirrup) as eardrum moves hammer, hammer moves anvil, anvil moves stirrup. This system of bones acts like a system of tiny levers which multiply the force of the vibration to the inner ear. Finally the vibrations are transported to the inner ear as the stirrup vibrates a tiny, liquid filled sack called the cochlea. The vibrations cause tiny hair-like sensory cells to move. This triggers nerve responses that send electrical pulses to the brain. Wow, this is an analog to digital converter!
This system is so well tuned that we can detect the direction of the incoming sound by the very small difference in arrival time from one ear to the other. That is, if the sound comes from the right, the right ear receives the waves a tiny bit earlier (and is a little bit louder) than the wave entering the left ear. Talk about good engineering!
When waves strike matter (like the eardrum), they can set the matter into vibration! This form of forced vibration can be seen when a tuning fork is struck. If the oscillating tuning fork is simply held by hand, the resulting sound may be rather weak. However, if the tuning fork is placed in contact with a pane of glass or table top, the vibrations are transferred to the second object, making it vibrate and amplifying the sound considerably.
Think back to our discussion on piezoelectric crystals. We found that they form the heart of an oscillator because the crystals vibrate with a natural frequency, and we try to keep it vibrating by feeding energy into the system at just the right time. Another example is seen when a child is playing on a swing and you add pushes at just the right time to keep the party going. The key is understanding that all matter has a natural vibration rate and you carefully time things by adding energy into the system at the right time and place to keep things moving.
One famous physics demonstration has a tuning fork on one side of the table (left). On the other side (right) are a variety of different tuning forks which include one that identical to the tuning fork at the left side of the table. Now strike the one fork on the left and guess what happens? Only the identical fork at the right is set into vibration. That is, only an identical tuning fork is capable of accepting these waves and it starts to vibrate itself. This is resonance. Click link 6.2.c for a neat demonstration
Resonance or "sympathetic vibrations"
Since each piano string has its own resonance frequency, you can demonstrate this yourself if you have a piano at home. Open the top and scream into the wires. You will soon hear your scream in the form of vibrating strings. Try high pitched screams as well as low pitched screams to convince yourself that there is a very real connection between matter and waves.
You bet it can, and it is the key to understanding all wind instruments (trombone, trumpet, flute, etc.).
When we studied lasers, we introduced you to the Principle of Wave Superposition. That is, waves can interfere constructively or destructively. In the case of sound, if two compression waves meet, they add together to make an even louder sound. If a wave compression meets up with a wave rarefaction, the two cancel each other out and you get silence.
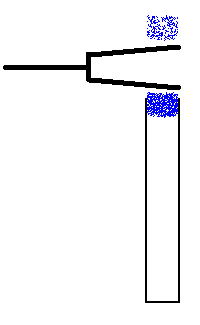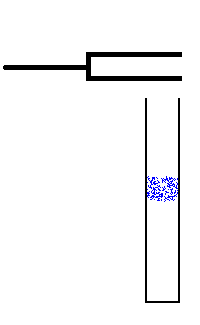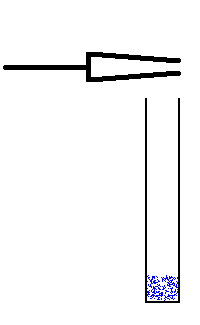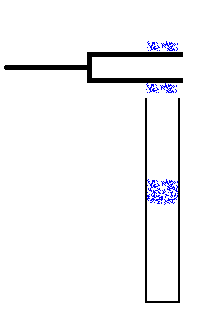
Another classic physics demonstration (animation)
The key to seeing how wind instruments work is demonstrated in a classic physics experiment. A tuning fork is set in motion over a cylinder which is open at the top and closed at the bottom. Water is slowly added to the cylinder and at a certain point, a very loud sound is heard. At this point, the system is "tuned" so that a compression (which moves down the tube and bounces off the water) meets constructively with next compression and an amplified sound is produced. It should be clear that sound waves with lower frequencies will require a longer path to produce the same effect. Wind instruments work the same way. A reed or fipple (mouthpiece) is usually used to create a vibration within an air column. Air moves in patterns to create constructive interference at the exit point. Click link 6.2.d for a demonstration
A speaker converts electric pulses to mechanical vibrations, which make sounds. The key is an electromagnet surrounded by a permanent magnet. When a varying current (which can come from a microphone) activates the electromagnet, the magnetic field it produces interacts with the permanent magnet, and the entire system vibrates. The rapid vibrations of this electromagnet set up vibrations in the speaker which then produces the sound. The speaker cone is designed so that different points from the center will resonate at different frequencies. That is, the cone will vibrate at lower frequencies (producing a lower pitched sound) near the outer rim and the higher pitched sounds come from the inner sections of the cone.
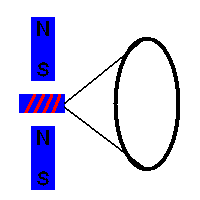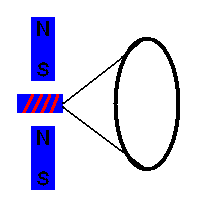
a speaker in action (animation)
If interested, click
link 6.2.e to see (and hear) a
cool speaker demonstration.
If interested, click
link 6.2.f to read an article about speakers.
Certain crystals and ceramics can convert electric signals directly into mechanical vibrations using the piezoelectric effect. The vibrations of the crystal or ceramic then generate sound waves. This is how a simple buzzer works. You most likely have this kind of a buzzer in your smoke alarm.
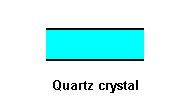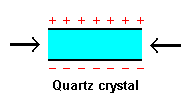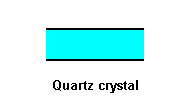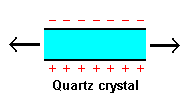
A Piezoelectric buzzer (animation)
The horn on your car uses an electromagnetic and a plunger (remember the solenoid?) to initiate the vibrations. However, when the plunger is drawn into the electromagnet, a switch breaks the flow of current in the circuit. A recoil spring retracts the plunger from the coil and returns it to the original position, the switch is closed again, the solenoid is drawn back into the coil, ...and the process repeats (thousands of times each second).
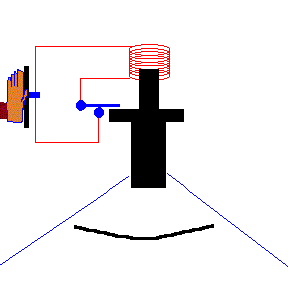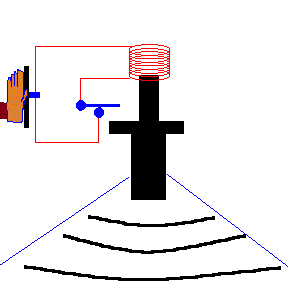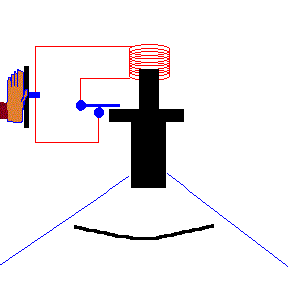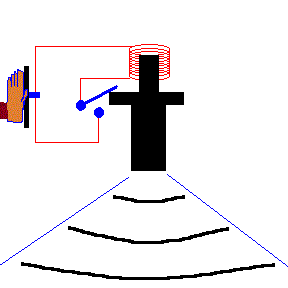
A car horn is nothing more than an on/off solenoid (animation)
You can click link 6.2.e to see a flash animation of a horn at work in slow motion (even though they call it a buzzer)
Can you reverse the process? That is, can you convert sound vibrations into electrical signals? You surely can! These are called microphones, and there are several ways to do the job.
One of the simplest microphone/ speaker systems consists of two tin cans attached by a tight string. Your voice makes one can vibrate, and the signal moves down the tight string. The second tin can converts the vibrations back to sound waves. However, no electricity is used in this process and this system is primitive, but it is a great way to learn science as a kid!
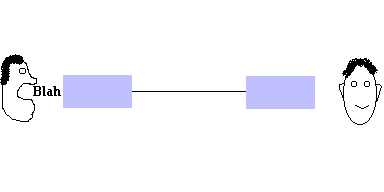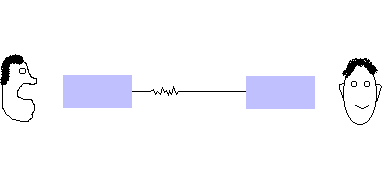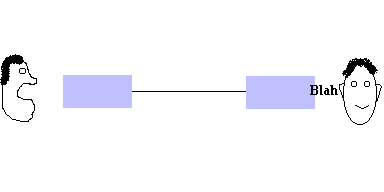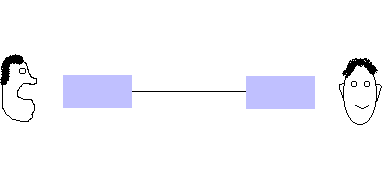
Did you make something like this as a kid? (animation)
The first electronic microphones used very fine carbon granules (almost in a powder form) which were confined between two plates. When a person spoke into the microphone, a diaphragm (like a balloon) would make these carbon granules vibrate and concentrate (or spread out) according to the sound. This arrangement was part of an electric circuit so that the electric resistance would vary according to the concentrations of granules. This, in turn, would vary the electric signal.
It is also possible to induce currents in a wire suspended in a magnetic field if the wire can be made to move across the magnetic field lines (remember electromagnetic induction?) A diaphragm picks up the sound vibrations and in turn, moves the wire.
You can even make a microphone using the Piezoelectric Effect. A vibrating diaphragm attached to a crystal or ceramic will generate electric pulses. This is how the stylus (needle) of an old phonograph record would convert your parent's rock-n-roll records into sounds.
|
Moving coil microphone (animation) |
Piezoelectric microphone (animation) |
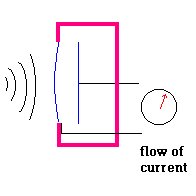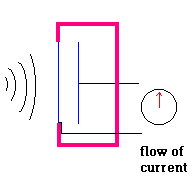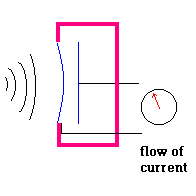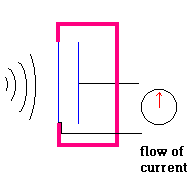
A simple capacitor microphone (animation)
You can even use capacitors to make a microphone. One of the capacitor plates is attached to the diaphragm, and the other remains fixed. Once a capacitor is charged up, the voltage it holds depends on the separation of the plates. If the plates move apart, the voltage increases ... if the plates move closer together, the voltage decreases. Again, the secret is a diaphragm which vibrates according to the sounds it receives. Thus, an electric current is set up as a result of sound vibrations.
A telephone system is now very easy to understand. It is nothing more than a microphone on your phone sending a signal to a speaker on the other persons phone..
Sound is strictly a compression wave. The earth produces waves too. When tectonic plates move, they store a lot of electric energy in brittle rocks near the surface. If the stress in the rock is too great, the rock fractures, producing a fault. This also releases a lot of energy in the form of seismic waves in an event commonly called an earthquake. Earthquakes emit two completely different forms of wave - compression waves and transverse waves. These events also offer us a chance to clarify the difference between sound (a compression wave) and light (a transverse wave). In a compression wave, the displacement of the medium is in the same direction as the direction of the wave itself. A transverse wave produces a displacement in the medium which is perpendicular (right angles) to the direction of the wave motion. The next time you are at an arena, the crowd might "do the wave". The hands go up and down but the wave travels left or right. You become part of a transverse wave. Go Badgers! Link 6.2.f
Geologists have determined that there are two distinct vibrations that occur during an earthquake:
|
Compression Waves (P wave animation) |
Transverse Waves (S wave animation) |
These waves travel through the earth with different speeds. They
also bend (refract) as they move, depending on the nature of the material
they move through. P waves also known as primary waves or
compression waves, create disturbances in the same direction in which they
propagate, just like sound. A good example of a "P" wave is the motion you would expect if
one car rear-ends another car ... and that car, in turn, rear-ends the car in
front of them, etc. S waves (also called shear, transverse,
or secondary waves) create disturbances at right angles to the direction in
which they propagate, so is light. "S" waves do not go through liquids (one
way we know the outer core is liquid). When an earthquake occurs,
geologists use the data from seismic stations across the planet to map the
interior of the earth.
During thunderstorms, one quick way of estimating the distance to a lightning strike is to count the time between the visible flash and the thunder. Since light travels almost instantaneously and sound at about 1000 feet per second, you can estimate distance by measuring time differences. The rule of thumb, here, is: 5 seconds delay = 1 mile. That is, if you count 15 seconds between the flash and the thunder, the strike occurred 3 miles away. The same idea can be used with seismic waves to determine how far away the epicenter is from your station.
Geologists use seismographs to record and measure earthquake activity. It basically relies on inertia as the instrument shakes (along with the building), while the heavy plumb remains motionless.
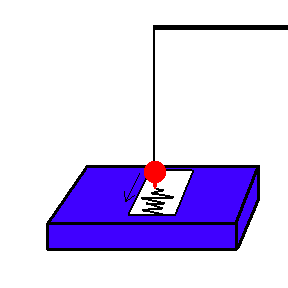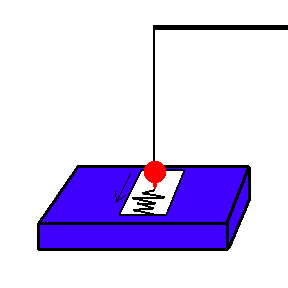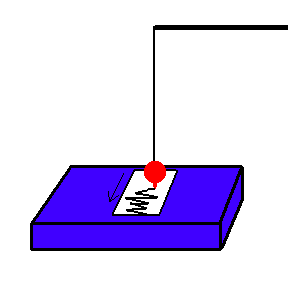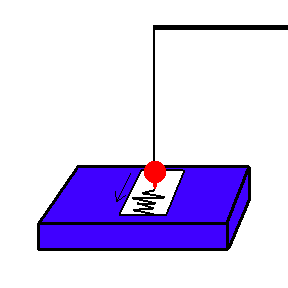
How a seismograph works (animation)
Any given station is able to tell how far away the epicenter is by relying on the fact that the "S waves" and "P waves" travel at different rates.
Speed of "P Wave" = 6 km/sec
Speed of "S Wave" = 3.5 km/sec
Difference in speed = 2.5 km/sec
The farther you are from the epicenter, the greater the time gap between the arrival of the P wave and the S wave.
For example, if there is a 50 second difference between the arrival of the S waves and P waves, you would know (from the chart) that the epicenter was approximately 500 km away.
At least three seismographic stations are needed to pinpoint the location of an earthquake. By using a method of triangulation, three seismographic stations can tell where an earthquake occurs. They can also estimate the magnitude (Richter scale) based on the intensity of the "S Wave" (and an understanding of how it decreases with distance).
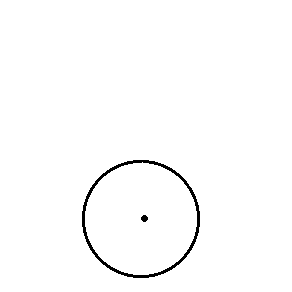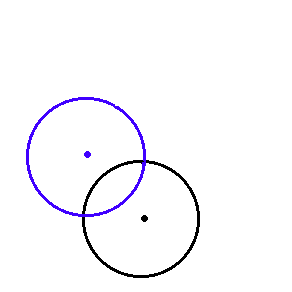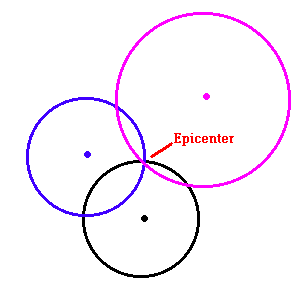
Finding the epicenter requires data from 3 stations (animation).
We can apply the same idea to GPS receivers. If you are willing to ignore the fact that GPS systems use electromagnetic waves (a transverse wave) in a unit dedicated to sound (a compression wave), we can extend the idea presented above to see how a GPS receiver can pinpoint your location on a globe.
Introduction - The US defense department has 24 satellites orbiting the earth so that at any one time, from any point on the earth, your GPS receiver can receive signals from at least 4 orbiters. These satellites orbit at about 12,000 miles and each one transmits a coded pattern your GPS receiver recognizes. How this system works can be broken down to 3 steps.
Step #1 - Pinpointing the location of 3 satellites. Before your GPS receiver can tell you where YOU are standing, it needs to know where each satellite is. Your GPS receiver holds all the information about the orbits of these satellites. If it knows the exact time, it knows the precise location of all 24 orbiters. As an analogy, if you give an astronomer the exact time, he/she could tell you the precise location of the moon since all the orbital properties of the moon are known.
Step #2 - Find the distance to 3 satellites. Each satellite has an atomic clock which is accurate to the nanosecond. It uses this clock to send out a coded signal at regular intervals. At the same time, your portable unit replicates the same coded signal. Since light travels at 186,282.397 miles per second, the hand held unit receives the satellite signal with a small time delay (compared to its own clock). This is converted to a distance (distance = rate*time). If you followed the logic used by seismologists to find the epicenter, this form of triangulation is just slightly more complicated. Since we are dealing in 3 dimensional space, we are working with spheres (not circles). That is, if you calculate a distance of 13,000 miles to a satellite, you could be anywhere on a sphere, 13,000 miles from the satellite. If you now find the distance to a second satellite, the intersection of the two spheres is a circle. The distance to a third satellite limits your possible locations to two points in space. See the animation below.
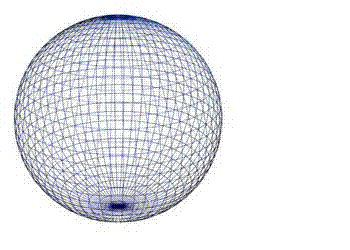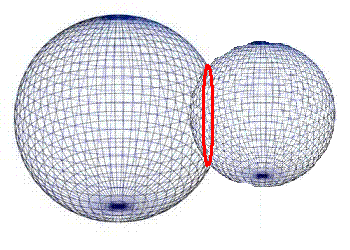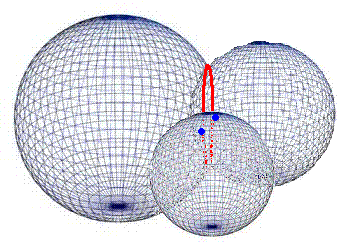
Triangulating 3 spheres offers two solutions (animation).
One of these solutions is ignored because it places you far above the earth's surface. This means the signal from just 3 satellites has pinpointed you somewhere on the surface of the earth. The GPS display will show your exact latitude and longitude.
Step #3 - The need for a fourth satellite. This step is a bit tricky so try to hang with me. The technique listed above assumes that the handheld GPS receiver is set with atomic clock accuracy. It is not. Once set, it will run with high precision, the problem is, ...what is the initial setting? This is accomplished with the help of a 4th satellite. Let's assume the handheld receiver's clock is in perfect sync with the atomic clocks on board the 3 transmitting satellites. If so, the data received by the 4th satellite offers a confirmation check. That is, it should confirm that the user is at the exact position the display reads. If it doesn't check, it knows that there must be an error in the setting of the handheld clock (it is NOT in sync with the satellites). The receiver then works the problem backwards. It resets itself to a different time and recalculates position. It then uses the 4th satellite to confirm the location. If it checks, you know the timer is set correctly and the location is accurate. If not, another time reset is required. This keeps going until the data from the 4th satellite offers a confirmation.
If interested, click link 6.2.g to read more about GPS systems.
©2001, 2004, 2007, 2009, 2016 by Jim Mihal - All rights reserved
No portion may be distributed without the expressed written permission of the author