courtesy NASA at http://grin.hq.nasa.gov/index.html
Are these astronauts weightless?
Gravity is the "glue" which holds the universe together. Later we will see that nature had given us other forces ... but it is gravity that covers the "big picture". In this section, we will see some of the ways gravity effects us.
In 1957, Russia launched the first artificial satellite, Sputnik. There are now over 1,000 operational crafts orbiting Earth (2013) ... and many thousands more which no longer work. Whenever you see an image from space, the astronauts are viewed floating around and is usually associated with the word "weightless" environment ... wrong!
|
Are these astronauts weightless? |
|
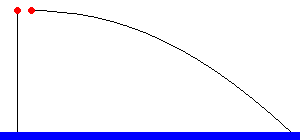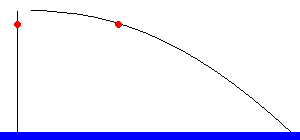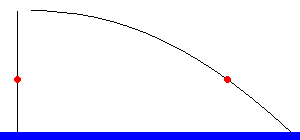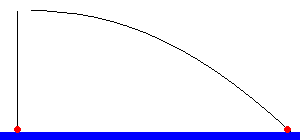
If you have ever taken a physics class, you learn the details about projectile motion. One of the most interesting demonstrations deals with this situation: If a person fires a bullet from a gun which is held horizontally and at the same time the gun fires, the person drops another bullet from the same height, which bullet hits the ground first? OK, I've never done this with a gun ... but using a similar setup, you will find that both bullets would hit the ground at the same time.
Projectile motion animation
The whole point is to show that the bullet fired from the gun is actually falling (due to gravity) in exactly the same way the bullet which is dropped from the hand. Of course, there are certain assumptions built into this demonstration ... that being that this experiment is done in a vacuum (no air friction) and that the ground is perfectly horizontal. Given that, the faster the projectile is fired, the farther it goes. The faster the projectile is fired, the path of the bullet shows less arc ... but it still hits the ground at the same time as the other bullet because it is always falling.

The faster the projectile, the less curve to the arc (animation)
So let's suppose you were able to fire the bullet at 17,500 miles per hour? The bullet would still fall, and still have an arc, but quite a special arc. You see, the arc it would take would exactly match the curvature of Earth. Our assumption that the ground is perfectly horizontal no longer applies and the bullet simply falls ... and falls ... and falls ... and never hits the ground!
Click here and shoot a cannon ball into orbit and you should get the idea that all satellites are in a perpetual state of freefall. Now think about an astronaut inside a satellite (like the International Space Station). That person is also falling (along with the ship). Of course they appear weightless but then again, so would you if you were in an elevator and the cables broke (don't try this, please). Physics folks call this situation a "zero g" environment which is a fancy way of saying they appear to be outside any gravitational field ... but of course, you now know that they are most definitely under the influence of gravity (and as a result, have weight). Now it is true that those same astronauts are farther from the earth's center and because of the inverse square law, they weigh less, but they are not weightless.
To put a rocket or shuttle in orbit, all you need to do is get it above the atmosphere (so there is no friction to slow it down which is about 100 mile above the surface) ... get it moving at 17,500 mph (5 miles per second) sideways ... and shut off the engines. This low altitude orbit means the payload will take about 90 minutes to orbit Earth. If you are interested, you can go to this site, and it will tell you exactly when satellites (like the International Space Station - ISS) will appear over your location.
If you place a satellite much farther from Earth ... just over 22,000 miles, its orbital period is one day. Place that same satellite over the equator and it appears to be "fixed" over one location (because it now orbits with the same period as the earth's rotation). This is known as a geosynchronous orbit (or geostationary orbit) Satellites in these orbits are useful for communications and imaging (watch any weather image on TV).
|
animation |
|
Credit NASA
Astronomers have to use every tool at their disposal to learn about our universe ... and gravity can be one very handy tool. Newton tells us that for every action, there is an equal and opposite reaction. That is, if "A" pulls on "B", then "B" pulls back on "A". Another thing Newton tells us is that every object in this universe exerts gravitational forces on every other object in the universe. Of course, the size of that force depends on mass and distance (inverse square law). Students often ask if global catastrophes can occur when planets line up (due to gravitational influences). Although the mass of planets may sound like a lot, at their current distances, the overall gravitational effect is miniscule ... not enough to lose sleep over. But it is not zero! In 1845, two young astronomers working independently, Adams and Le Verrier, had noticed that the orbit of Uranus had deviated slightly from the path predicted by Newton's equations. They both surmised that an undiscovered planet was responsible for this effect. Working backwards, they calculated the location this planet had to be to produce these perturbing effects. The next year Neptune was found exactly where each had predicted. This was a triumph for Newton's theory, and both Adams and Le Verrier are given credit for discovering Neptune.
Planet 9 ???? ... from outer space (sorry ... my bad)
Ok, it has not been found ... yet. However, history repeats itself when astronomers announced (on 1/20/2016) that an unseen planet could be the reason several Kuiper objects are "clustering" in an unexplainable manner. Computer models indicate that the gravitational tug of a surmised "Planet 9" could solve this mystery. Planet 9 would have to be very massive (about 10 times the mass of Earth) and very far away (600 AU). Observing such a planet is currently beyond our technology. It would be far too dim in the visible spectrum to be seen (since it reflects sunlight). Perhaps it could be discovered by its own "heat signature" in the far infrared. Anyway, there is nothing official ... so we are still holding at 8 planets in our solar system. Stay tuned.
So now you have an idea what a "perturbation" is. It is a slight deviation in the motion of an object due to gravitational forces exerted by another object. Now study the animation below carefully.
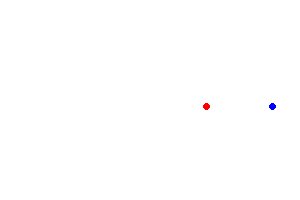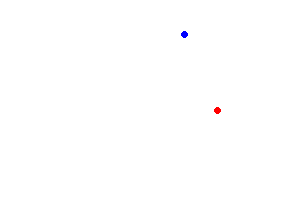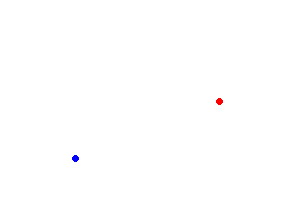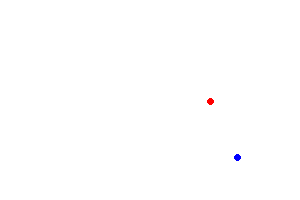
Two objects in a gravitational dance (animation)
Extrasolar Planets (Exoplanets)
As you watch this animation, assume you are watching two astronomical objects in orbit. You can clearly tell that the red object has much more mass than the blue object (a good astronomer could tell you the exact ratio) by observing the degree of influence one object has over the other (how much one object can perturb the other). It should be clear that one object doesn't simply orbit the other, they both orbit around a common point (known as the barycenter). Now assume you are colorblind in blue and can only observe the red object. Physics would have to tell you that there is an "invisible" companion perturbing the red object ... and a very clever person could even tell you its orbital properties (such as sidereal period and semi major axis). This is exactly how astronomers are able to make claims that planets orbit around other stars. There are several different techniques used to discover extrasolar planets. However, most of the first extrasolar planets were found by observing the perturbations (wobble) they produce on the star they orbit. We will discuss other ways to find exoplanets in the next unit. Click here to see the current list of extrasolar planets (which is well over 1000 confirmed planets by 2014).
The majority of extrasolar planets found with this "wobble method" were not like anything we see in our solar system. Basically they are "Hot Jupiters" ... meaning they are very massive planets which orbit very close to the star they revolve around. Does that mean our solar system is the "odd ball"? Not necessarily! Which extrasolar planetary systems are most likely to be detected (with this method) the easiest -systems with low mass planets or high mass planets? Answer: High mass ... because they produce the stronger gravitational effect on the star they orbit. Which extrasolar planetary systems (with this method) are most likely to be detected the easiest - planets which orbit close to their sun or which orbit farther from their sun? Answer: Closer ... because gravity gets stronger the closer you are. So you can see that if you are finding planets by observing the gravitational "wobble" or perturbations they exert on the star they revolve around, this technique is biased toward finding "Hot Jupiters". Our solar system may very well be quite "normal" ... we may have to wait a few decades to answer that question. The good news ... several of the other detection techniques do not have this bias built in so we may soon learn what a "typical" planetary system around other stars looks like.
We will have to wait a while before we cover black holes (and their properties), but there is very little doubt that they do indeed exist. Why? Go back to the animation above, and this time imagine you are colorblind in red. Watching the motion of the blue object may convince you that there must be a much more massive object somewhere exerting a powerful force on it. Astronomers have found stars moving in orbits around "invisible" companions. Their calculations have convinced them that black holes are the only conceivable answer. Stay tuned for more ...
Johannes Kepler discovered all his laws by observation. If you recall, his 3rd law was written as: P2 = a3 where a was average distance to the sun (semi-major axis)... in AU and P was sidereal period .. in Earth years. It worked but Kepler himself had no idea why. Then came Isaac Newton and his law of gravity. Using theory, Newton was able to derive the "true" form of this law ... which showed that Kepler left something out ... mass. The actual relationship turns out to look like this:
(msun + mplanet) P2 = k a3
Where msun & mplanet represent the total mass of the system and k is a constant. So why did Kepler miss this term? If you understand that almost all the mass in our solar system rests in one object ... the sun, then this term really doesn't show any variation when you apply it from one planet to another within our solar system (so don't blame Kepler for not finding it). However, this "revised" form becomes important for astronomers because it now gives them the ability to find the (total) mass in any 2-body system which is bound by gravity. Let's use a more generic form below so we can apply it to any two masses in a gravitational dance.
(m1 + m2) P2 = k a3
Suppose you wanted to find the mass of the planet Mars, for example. In 1877, two very small moons, Phobos and Deimos, were discovered orbiting Mars which allowed you to make that calculation possible (actually you only need to find one small moon). By examining the orbit of one of these small moons, astronomers quickly found both the average distance, a, and sidereal period, P. Anyone who has taken an algebra class knows that if you have an equation with 3 unknowns and are given values for two of the variables, the third value can be determined. In this case you could find (m1 + m2) which is the total mass of the system ... Mars plus its moon. Since the moon's mass was so much less than the mass of Mars, it washes out ... leaving the mass of Mars. The planet Venus has no natural satellite so we had to wait much longer to find its mass. That occurred when we placed probes in orbit around Venus in the 1960's. This same technique allows astronomers to determine the mass of most stars .... because more than half of all stars are found in a binary system (stars orbiting other stars).
You can't fell the difference, but the pull of gravity at your head is slightly (and I do mean slightly) weaker than the pull of gravity on your feet. That is because your head is further away from the center of Earth than your feet (remember the inverse square law). The difference is so little because you might be only 6 feet tall and that extra distance is insignificant when you consider the radius of Earth is about 4,000 miles. That all changes if you were considerably taller (say 1,000 miles tall). Now the difference would be quite noticeable and the effect we would call "tidal forces". Think of this example as we now discuss tides.
Check out my Google Presentations on tides - click here

Basic idea behind tides
Because the strength of gravity gets weaker with distance leads to an interesting phenomena when masses interact. They tend to get stretched!!!! The animation above is intended to show the effect known as "tidal forces". It is not so much how strong the overall force of gravity is, but rather, how much stronger that force is on one side of the object compared to the other side. Allow me to explain this statement. Consider two masses close to each other as in the image below.

The presence of the nearby (black) mass exerts a gravitational force on the other (blue) mass. However, because gravity gets weaker with distance, the force at point A is stronger than at location B. Since location C is even further away, the force of gravity there is even weaker yet. This results in a 7% stronger gravitational force at point A compared to the far side at point C. Without getting too technical, the result of this effect is the body gets stretched. Maybe this picture will help you see why there is a stretching effect in case you are interested.

This is exactly what happens in the Earth-Moon system. Again, without getting too technical, the presence of the moon compels Earth to assume this shape .... only .... since the surface of Earth is covered (mostly) with water ..... and water is very easy to manipulate ..... it is the water that plays the major role here. The result is a bulge of water on BOTH sides of Earth .... one facing the moon and another on the "back side". Don't worry, your job is to accept this concept on face value and move on.

Now put yourself at point A and you are at "low tide". In 6 hours, the rotation of Earth moves you to point B and you experience a "high tide". Six hours later, low tide, etc. Ok, it isn't exactly 6 hours because the moon moves as well, but you get the idea.
Some interesting effects of tides
Tides can produce some very interesting effects on our planet. One is known as "tidal braking". Think of the water bulge as a more-or-less permanent feature that is always lined up with the moon. The rotating solid Earth beneath this bulge (seabed) is constantly creating friction with the water (much like a car's drum brake is used to slow the car). This friction is very gradually slowing down the rotation rate of Earth. You will never notice that a day is getting .0023 seconds longer every century as a result of tidal braking ... but the effect can be considerable over billions of years. The early Earth had a much shorter day/night cycle.
The laws of physics will not allow this loss of angular momentum without some compensation elsewhere. The loss to Earth results in a gain to the moon. This is an obscure way of saying that because of tidal braking, the moon is slowly spiraling away from us. Every year the moon moves 3.8 cm further away. Billions of years ago, the day was much shorter AND the moon was considerably closer (bigger in the sky). In these days, tides were much higher than we see today!
Let's not forget that Earth produces tidal effect on the moon as well. During early times in the history of the solar system, a much more geologically active moon underwent a kind of tidal braking as well. The moon never had liquid oceans of water but it did experience tidal braking when it did have periods of molten rock. This slowed the rotation rate of the moon to the point where it acquired a tidal lock with Earth. This is a fancy way of saying that one side is always facing Earth and the back side is hidden from our view.
Bring in the Sun
Even in grade school you learned that the tides are caused by the moon, but the sun plays a role in this discussion as well. The solar effect is considerably weaker than the lunar effect (much less stretching) but things get interesting when the solar effect reinforces the lunar effects OR when they work against each other.

Spring Tides occur when the tidal stretching effects of the sun work WITH the tidal stretching effects of the moon. It has nothing to do with a season but, rather, a time when tides "spring up" to extreme heights. High tides are really high and low tides are really low. The animation shows that this will happen during a new moon. However, since we are only dealing with stretching effects in the horizontal direction, it should be obvious that spring tides will also occur during times of a full moon as well (since the "bulge" occurs on both sides of Earth).
On rare occasions, we experience a "supermoon". This
occurs when the moon is full AND it coincides with the perigee (closest to Earth in its elliptical path). You can expect "super tides" at this time
as well (a term I made up) .... actually it is called a perigean spring tide.
I experienced this in Florida a few years back. The high tides were higher
than I've ever seen them and the low tides freaked people out. We could
walk to some islands we normally kayak to.
Can nature create the perfect storm? You bet! Imagine a hurricane is moving in at the same time as a high tide. The low air pressure from the hurricane produces a much higher water level along with a strong storm surge inland. Combine that with a high tide ... or a high spring tide ... or worse yet, a high perigean spring tide and .... bad news. Ask the victims of Hurricane Sandy about this. At least the moon was near apogee at this time ... or it could have been even worse.
Neap Tides occur when the tidal stretching effects of the sun work AGAINST the tidal stretching effects of the moon. This occurs when one body works in the horizontal direction and the other works in the vertical direction ... partially canceling each other out. During neap tides, high tides aren't so high and low tides are not that low. You might be able to guess the moon phases associated with neap tides - first and third quarter phases of the moon.
Other Tidal Effects

Io, the pizza moon
Volcanoes on Io - One of my favorite moons in the solar system is the volcanic moon, Io, orbiting Jupiter. Every day Io resurfaces itself with lava ... but where does all this internal heat come from?? Io orbits very close to Jupiter and the "tidal effects" on Io are extreme. These tidal forces stretch Io, but the dynamics of its orbit mean that Io is stretched from different directions (with respect to a fixed point on the surface). This means Io is physically molded into a different shape on an ongoing basis. This produces internal heat which must escape to the surface in the form of volcanoes. In an analogy, think how your car tires are constantly reshaped as you drive down the road ... making the tires hot.

Comet Shoemaker-levy 9 - When this comet was discovered, it had already suffered a catastrophe. This comet got too close to Jupiter and the tidal forces were so great that it ripped the comet apart into 21 individual pieces. Maybe even more interesting was witnessing each fragment plunge into Jupiter in 1994 .... the first time anyone witnessed such an event.
A little moon is getting stretched - Phobos orbits very close to Mars .... maybe a bit too close. It is showing signs of being stretched by tidal forces. The situation will get worse as the orbit of Phobos spirals closer and closer to Mars. Eventually Phobos will get pulled apart, possibly leaving Mars with a ring of debris.

Stretch marks on Phobos (Credit NASA)
Stay away from Black Holes - A discussion of these bizarre creatures is yet to come, however, this is a good time to answer one question. What happens when you fall into a black hole? This is a loaded question because if you were unfortunate enough to approach a black hole, the tidal forces would make the question mute. Just as Shoemaker-levy 9 got ripped apart, so too, would you. The effects would be so extreme you would be stretched apart on the atomic level. Astronomers have even invented a word to describe this extreme tidal effect - spaghettification .... meaning you will be pulled apart like spaghetti.

Tidal Forces on Galaxies - Sometimes two galaxies get too close to each other and .... well .... guess what? Tidal forces can stretch them out of shape. A good example is the Tadpole Galaxy shown below which has a "tidal tail"

ŠJim Mihal 2004, 2014- all rights reserved