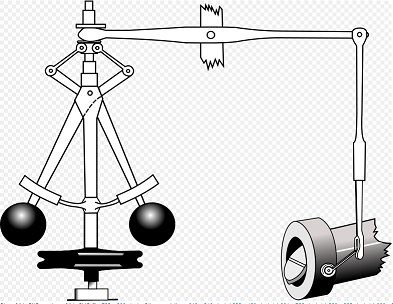
Watt
Centrifugal Governor Courtesy
Wikimedia Commons
... or putting a new spin on things. You have heard the phrase "spinning out of control". In fact, you can GAIN control by the action of a spinning object.
Key Terms
centrifugal force
centrifugal governor
centrifuge
centripetal acceleration
centripetal force
feedback (negative & positive)
geosynchronous orbit
inertial switch
moment of inertia
Centrifugal Governors ... the classic controlling device
The governor is a component in an engine which maintains a certain RPM based on the setting of your throttle. If your throttle is set for a certain speed (say 1000 RPM) and you place a load on the engine which begins to slow it down, the governor will compensate by opening the carburetor and allowing extra gas and air in. Most tractors and small engines (like on your lawnmower) have a governor between the throttle and the carburetor.
Below is a picture of an early governor developed by James Watt in his steam engine

Watt
Centrifugal Governor Courtesy
Wikimedia Commons
As the flywheel slows in rotational speed, the arms lower. This opens the throttle valve to release more steam Ö making the device spin faster. Likewise, if the governor spins too fast, the arms rise up to close the throttle valve. This cuts the steam supply and the device spins at a slower rate. This makes the governor a perfect feedback mechanism to keep the shaft spinning at a nearly constant rate.
A similar device is found in elevators. If there was a problem and the car suddenly accelerates beyond safety limits ... a centrifugal governor activates a safety brake. Some rental trucks and school buses have these devices built in to avoid excess speeds.
Some small engines (50 cc and under) have a device that limits the RPMs in a similar manner. They have a small device that is linked to the throttle that is activated by air blown off the flywheel. If the RPMs rise too high, the flywheel spins faster and makes more draft ... which in turn pushes on a small "sail valve" which turns down the mixture to the carburetor. Modern engines may control the RPMs by "electronically" counting the speed and sending the information to a microprocessor.
All cars need transmissions. This is because the engine spins at a small range of RPM but the wheels need a much larger range of speeds (and torque). There is a governor in the automatic transmission of your car which tells the system when to change gear ratios. As the rotation speed increases, the spring retracted governor will either allow more or less hydraulic fluid through the system to open/close bands and clutches ... allowing gears to spin at different rates and directions.
| What is feedback?
When you have a "cause-effect situation", the effect can produce an outcome condition which, in itself, either stimulates or retards repetition of the initial "situation". A positive feedback promotes an increase in activity and a negative feedback cuts the activity back. Example of POSITIVE feedback: Cold weather produces snow. Snow cover tends to reflect incoming sunlight back to space (rather than absorb it). This, in turn, tends to make the area colder ... and produces more snow. Example of NEGATIVE feedback: Sunlight on the ocean promotes evaporation. More water vapor in the air stimulates cloud formation. Clouds block incoming solar. This reduces evaporation from the ocean. As you can see, a positive feedback loop is a "run-away" situation which tips the balance to one extreme. A negative feedback loop tries to keep things in check. Many devices we talk about in this class (including the governor) try to do just that ... keep things in balance. As we look at other devices in this course, see if you can identify negative feedback in the design. |
Have you ever wondered what happens when you pull the rope to start your lawn mower? Just like the very first cars, you have to get the motor turning by hand to get it started. Although we will cover the way an engine works later in this class ... for now, let's just see how the manual starter works.
As you know, you have to pull on a rope and the engine turns as a result. But how does the starter engage the engine? The starter assembly attaches to the top of the engine's flywheel. When you pull on the rope, small "swing levers" jet out and engage cogs on the inside hub of the flywheel. Normally, these swing levers are held inward by small springs. Once you are done pulling on the rope (and the engine starts up), the swing levers retract to their rest position.
|
|
|
Similarly, my chain saw uses a centrifugal clutch to transfer the energy of the engine's spinning crankshaft to a drum (that moves the chain). As the engine revs up, friction plates move outward and make contact with the drum. At low engine speeds, retraction springs release this contact and the chain stops moving.
This same idea is used in a centrifugal switch. Some induction motors use an extra set of windings to kick-start the motor when it initially starts up (to overcome inertia). Once the motor reaches normal operational speed, power to these extra windings is no longer needed, so you need a way of switching it off. Can you see how the ideas used here can be used to break an electrical connection when the shaft is spinning at a certain speed? The next time you start up an electric motor, listen for a "click" soon after you flip the "on" switch, and you will understand what is happening.

Photo Copyright by Wayne Wooten (used with permission)
Why do spinning objects "fly outwards"?
Most people would say that these are examples of "centrifugal forces" ... implying that the objects swing out because there is a force pushing them away from the center. Wrong! These are examples of an objects inertia which ... according to Newton's First Law of Motion ... says that if an object is in motion, it will continue in motion ... in the same direction.
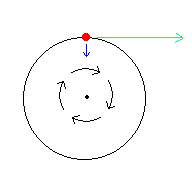
This diagram shows that if an object (shown as a red dot) is seen moving in a circle, the "natural" motion is to move tangent to the circle (shown as a green horizontal arrow to the right). There is no mysterious force moving it away from the circle. In fact, there has to be an inward force on the object to keep it from moving along the tangent line. This force is known as the centripetal force (shown as small blue arrow pointing toward the center). In the examples above, the centripetal force is provided by ropes (amusement ride) and springs (lawnmower), and is always directed toward the center of the circle.
If we take this one step further, we need to apply Newton's Second Law of Motion. Since a net force is constantly acting on a spinning object (toward the center), it also implies that it is accelerating. Physicists call this centripetal acceleration. An acceleration is typically associated with an object moving in a straight line but going faster or slower. This is an acceleration associated with a change in direction but it is an acceleration, none the less.
If you were to tie a rope to a rock and swing it in circles over your head, YOU would be providing the centripetal force on the rock as you strain to keep the rock in its course. Since Newton showed that forces always act in pairs (Action - Reaction), the rock would pull on you with an equal but opposite force. This would be a centrifugal force and has no bearing on the behavior of the rock since we are only concerned with the force acting ON the rock ... not forces provided BY the rock.
Question #1 - Would the centripetal force change if you replaced the rock with one more massive? (answers below)
Question #2 - Would the centripetal force change if you were to spin the rock faster (more rpm's)?
Inertial switches to avoid problems in a roll-over
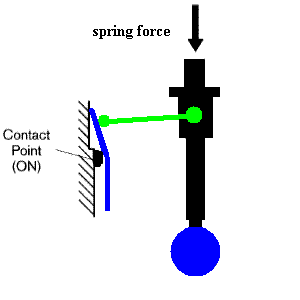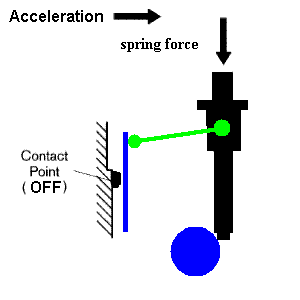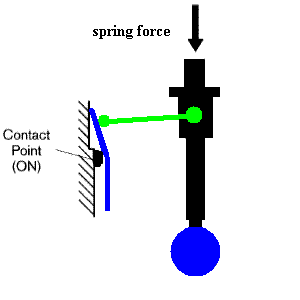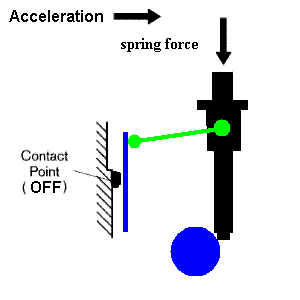
Inertial "kill switch" (animation)
Often it is essential to detect and/or limit the degree of acceleration in a system. This acceleration can be linear (straight line) or centripetal (going in circles). This can be controlled by an inertial switch, or other types of on/off switches. It may be something as simple as the tiny "swing levers" we illustrated above in the lawnmower. If the system is spinning too fast, a "swing lever" breaks an electrical contact and the system slows down. In the case of linear accelerations and/or rotational accelerations, an inertial switch (see animation) may be employed. For example, cars are equipped with these kinds of shut-off switches so the fuel pump shuts off in case of an accident (sudden deceleration) and/or a roll-over. The inertia of the metal ball resists the changes in velocity as the device is accelerated, and its separation from the support pin causes it to trip the switch if the acceleration exceeds a certain limit. This kind of switch is much like a circuit breaker. Once it is tripped, it needs to be manually reset.
In 1957, Russia launched the first artificial satellite to orbit the earth, Sputnik. There are several thousand crafts orbiting the earth today (most of which no longer work). Whenever you see an image from space, the astronauts floating around are usually referenced as being "weightless" ... wrong!

Are astronauts weightless? Courtesy NASA
If you have ever taken a physics class, you learn the details about projectile motion. One of the most interesting demonstrations deals with this situation: If a person fires a bullet from a gun which is held horizontally and at the same time the gun fires, the person drops another bullet from the same height, which bullet hits the ground first? OK, I've never done this with a gun ... but using a similar setup, you will find that both bullets would hit the ground at the same time.
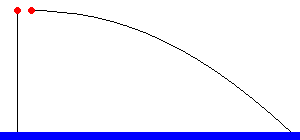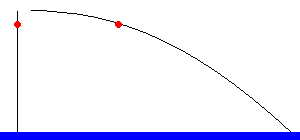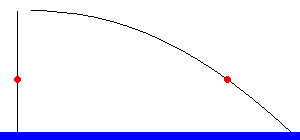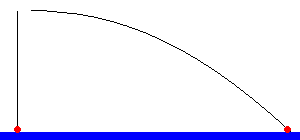
Projectile motion animation
The whole point is to show that the bullet fired from the gun is actually falling (due to gravity) in exactly the same way the bullet which is dropped from the hand. Of course, there are certain assumptions built into this demonstration ... this experiment is done in a vacuum (no air friction) and the ground is perfectly horizontal. Given that, the faster the projectile is fired, the farther it goes. As the projectile leaves the gun at faster and faster speeds, the bullet's path shows less and less arc ... but it still hits the ground at the same time as the other bullet because it is always falling. Keep in mind, the object is always under the influence of gravity (or it would fly off in a straight line). Click link 2.2.a for a video on this.

The faster the projectile, the less curvature to the arc (animation)
So let's suppose you were able to fire the bullet at 18,000 miles per hour? The bullet would still fall, and still have an arc, but quite a special arc. You see, the arc it would take would exactly match the curvature of the earth. Our assumption that the ground is perfectly horizontal no longer applies and the bullet simply falls ... and falls ... and falls ... and never hits the ground! We would say it is in orbit. The centripetal force comes from the gravitational attraction with the earth - i.e. weight.
Click link 2.2.b and shoot a cannon ball into orbit and you should get the idea that all satellites are in a perpetual state of freefall. Now think about an astronaut inside a satellite (like the International Space Station). That person is also falling (along with the ship). Of course they appear weightless but then again, so would you if you were in an elevator and the cables broke (don't try this, please). Physics folks call this situation a "zero g" environment which is a fancy way of saying they appear to be outside any gravitational field ... but of course, you now know that they are most definitely under the influence of gravity (and as a result, have weight). Now it is true that those same astronauts are farther from the earth's center and because gravity decreases with distance, they weigh slightly less. However, they are not weightless.
To put a rocket or shuttle in orbit, all you need to do is get it out of the atmosphere, about 100 miles above the surface, so there is no friction to slow it down ... get it moving at 18,000 mph sideways ... and shut off the engines. This low altitude orbit means the payload will take about 90 minutes to orbit the earth.
If you place a satellite much farther from the earth ... just over 22,000 miles, its orbital period is one day. Place that same satellite over the equator and it appears to be "fixed" over one location (because it now orbits with the same period as the earth's rotation). This is known as a geosynchronous orbit (or geostationary orbit). Satellites in these orbits are useful for communications and imaging (watch any weather image on TV).
|
Courtesy Wikimedia Commons |
Credit NASA |
Objects rotating about an axis (tops, flywheels, gyroscopes) are governed by laws of motion. There are many similarities between the physics of linear motion and the physics of rotational motion. Please spend a few minutes reviewing Newton's first two laws of motion presented in the last unit. You will recall that an object will tend to keep moving (or remain stationary) until a force acts on it. However, when a force does act on an object, it will accelerate. The rate of acceleration depends on the size of the force as well as the mass of the object itself. The more massive the object, the more difficult it becomes to change its state of motion. That is, you can think of "mass" as measure of inertia.
When we adopt these laws of motion to rotation, we must consider the net torque acting on an object (not just force). Objects in rotational motion tend to keep their state of rotational motion (or remain at rest) until acted upon by a net torque. When a net torque does act on an object, you can expect it to change its rotational speed. The rate of angular acceleration depends on the size of the torque. The larger the torque, the larger the expected change in angular speed. Now letís consider the mass (inertia) of the object. This is where you see one interesting difference between translational motion and angular motion. When we consider the rotational inertia of a spinning object, we need to consider the mass of the object as well as where that mass is distributed with respect to the axis of rotation. Physicists call this moment of inertia (I). You can think of moment of inertia as the "rotational inertia" of an object. That is, we replace the word "mass" (in the translational case) with "moment of inertia" (in the rotational case). Basically, this concept is trying to tell us that a spinning object becomes more resistant to changes in motion as the mass increases and/or the mass is distributed farther from the axis of rotation.
This quantity (moment of inertia) is a bit tricky to comprehend so let me demonstrate by example. Consider two tires (at rest) of equal mass but one is mounted on a 14" rim and another on a 16" rim. The tire mounted on the 16" rim has a higher moment of inertia (since the mass is farther from the axis of rotation). As a result, it has more "rotational inertia". If you apply the same torque to each tire, both will spin faster, but the tire mounted on the 16" rim will undergo a lower acceleration than the tire mounted on the 14" rim. You can think of the tire mounted on the 16" rim as being more resistant to changes in rotation motion. Now suppose both tires are spinning with identical angular speeds. Do you think each tire will have the same amounts of rotational kinetic energy? No! If you guessed that the tire mounted on the 16" rim has more rotational kinetic energy, you may be understanding the concept of moment of inertia. Hint: Recall that translational KE = Ĺ * m * v2 .. however, in the rotational case you need to consider I instead of m.
One interesting outcome of this is known as the "ice skater effect" (the actual name is the conservation of angular momentum). It deals in instances where an object changes its moment of inertia by altering where mass is distributed on a spinning object.
|
|
|
The ice skater effect (animation)
To help see how this works, consider an ice skater going into a spin. The skater has a certain amount of rotational kinetic energy (which we can assume remains fairly constant once they push off). The thing that the skater changes, however, is how their body mass is distributed in space. When the skater pulls their arms in close to the body, they spin faster. This action lowers their moment of inertia and as a result, they spin faster. In order to slow down, they redistribute their mass outward, increasing their moment of inertia. Physicists have found that a quantity called angular momentum (L) is conserved (does not change) during this process. Click link 2.2.c for a demonstration.
L = I x ω
where L is angular momentum, I is moment of inertia, and ω is angular velocity.
That is, product of the moment of inertia (I) and the angular velocity (ω) will remain constant. If one quantity goes up, the other goes down to compensate. When the skater extends their arms, I increases so ω decreases.
Answer #1 - Think about Newton's Laws! Specifically, F = ma. The centripetal force increases in direct proportion to the increased mass of the rock.
Answer #2 - Common sense should answer this one. It is the reason the centrifugal governor works in the first place. Mathematically, ar = v2 / r where ar is the centripetal acceleration, v is the linear speed and r is the radius of the circle. If the rock spins with more speed, acceleration increases and so does the force (F=ma).
©2001, 2004, 2007, 2009, 2016 by Jim Mihal - All rights reserved
No portion may be distributed without the expressed written permission of the author